Tool balancing and RPM

Increasing cutting speeds in combination with higher balancing requirements result in tighter balancing conditions for the total tool systems – machine spindle, clamping device and tool system.

Tool balancing requirements

Balancing according to ISO 1940-1 is often being intensified by additionally choosing the next better balancing grade (e.g. G 2.5 instead of G 6.3). Not only that this is technically often not necessary and leading to high costs it also cannot be achieved in many cases.

Another point of consideration to qualify the spindle load due to unbalance is that dynamic cutting forces (e.g. caused by the interrupted cut of a milling cutter) often are remarkably higher than the centrifugal forces caused by demanded permissible residual unbalances. The unbalance acts as a speed-harmonic excitation of the machine structure and the amount of the excited centrifugal force arises from the unbalance and the rotational speed.

The tool balancing quality requirements for rigid rotors stated in ISO 1940-1 (e.g. electromotor rotors etc.) cannot be applied appropriately to these total systems because machine spindles, clamping devices and tools show essentially different features:

• Machine tool spindles, clamping devices and tools are varying systems (e.g. by tool changes in machining centres)
• Due to radial and angular clamping inaccuracies a repeated tool change within the spindle leads to varying balancing conditions for total systems
• Fit tolerances of the individual components (spindle, clamping device and tool) set limits to the balancing process

Clamping inaccuracies between tool system and machine tool spindle set limits to the repeatability of the balancing conditions and in view of this, the balancing requirements of rotating tool systems have to take all essential parameters into account. The main objective is the limitation of unbalance related machine vibrations and system loads as well as process interferences.

The above circumstances set a new approach to specify the requirements for the balancing of rotating tool systems. The ISO 16084 standard content requirement of balancing rotating tool systems in a way that takes the actual load on the spindle bearings caused by the tool unbalance into account.

ISO 16084 specifies that the bearing load caused by unbalancing must not exceed 1% of the bearings dynamic load capacity. In this standard, all permissible residual unbalances are indicated in (g.mm) and are not assigned to a specific G-class quality level according to ISO 1940-1 - Balance quality requirements for rotors in a constant (rigid) state.

Tool balancing theory

Rotor unbalances can be caused by design, material, manufacturing and assembly. Every rotor has an individual unbalance distribution along its length, even in a series production.

Balancing is a procedure by which the mass distribution of a rotor is checked and, if necessary, adjusted. This is done to ensure that the unbalance force acting on the spindle bearings at the service speed is within the bearings capacity. The vibration of the journals is also controlled to be within the specified frequency limits.

Unbalance, U (g.mm)

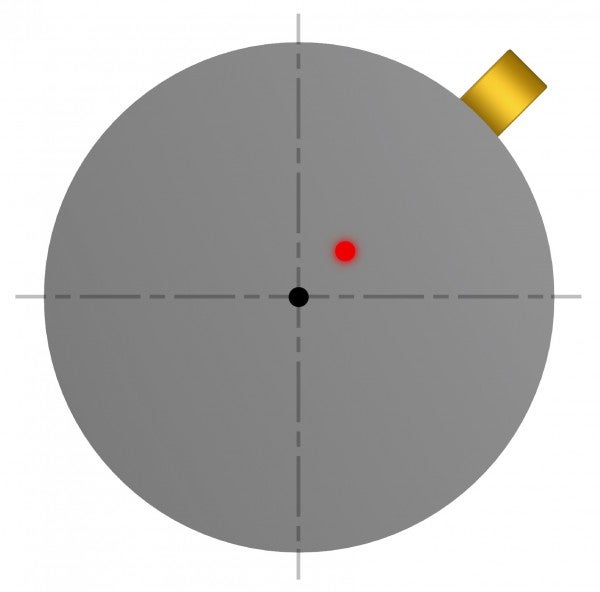
Is a measure specifying how much unsymmetrical distributed mass deviates radially from the rotating axis, see image below.

U = m × e

Eccentricity, e (µm)

The distance between the rotational centre and the centre of gravity, see image below.

e = U / m

Unbalance force, F (N)

The unbalance creates a centrifugal force which increases linear with the unbalance and squared with the number of revolutions, see centrifugal force in image below.

F = U × ω²

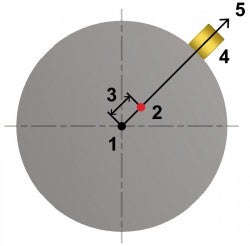
Tool balancing theory

1. Rotating axis
2. Centre of gravity
3. Eccentricity
4. Unsymmetrical distributed mass
5. Centrifugal force

Counterbalance

To compensate unwanted centrifugal forces, it is possible to remove or add material which assists in directing the centre of gravity to the rotating axis, see images below.

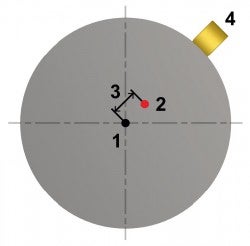
Unbalance

1. Rotating axis
2. Centre of gravity
3. Eccentricity
4. Unsymmetrical distributed mass

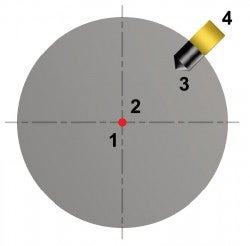
Counterbalanced with drilled compensating hole

1. Rotating axis
2. Centre of gravity
3. Drill compensating hole
4. Unsymmetrical distributed mass

Balance class according to ISO 1940-1 (G)

When balancing a tool to ISO 1940-1 balancing class G 2.5 at 20 000 rpm it is allowed to have an unbalance at 1 g.mm/kg (e=1 µm), see chart below. As an example a small Sandvik Coromant sticker corresponds to 4 g.mm. The ISO 1940/1 standard allows more unbalance on a heavier tool holder then on a lighter one at the very same rotational speed. This even though different unbalance creates different unbalance forces whereby no consistent system load is achieved.

According to ISO 1940-1 Balance quality requirements for rotors in a constant (rigid) state, G is a measure of the balancing quality of a rotating body. G is the tangential velocity at the centre of gravity in relation to the rotational speed. G is depending of the rotational speed (n), the body mass (m) and the unbalance (U). The G class is unidentified without knowing the rotational speed.

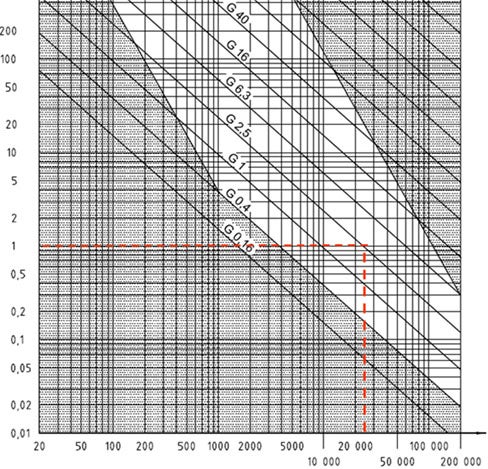
Permissible residual specific unbalance for different ISO 1940-1 G

X-axis: Service speed n, rev/min

Y-axis: Permissible residual specific unbalance, e_per, g.mm/kg

Calculation example ISO 1940-1

Coromant Capto® C4 tool holder

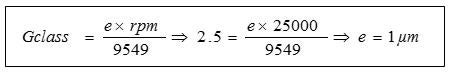
• Balance class: G2.5 at 20000 rpm
• Tool mass: 1.0 kg
• Balance class equation
• Eccentricity = Unbalance/mass of tool

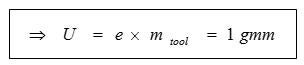
Sandvik Coromant sticker corresponds to 4 g.mm

A standard tailor made for the application of high speed cutting tools. The ISO 16084 standard means that the rotor tool system is balanced in a way that takes into account the actual load that the spindle bearings are subjected to, does not use the different G-classes but instead gives a specific value of the accepted unbalance (U) for every tool in g.mm.

As the ISO 16084 is tailored just for the application of high speed cutting tools, this also implies that a series of new parameters compared to the old ISO standard is introduced. This allows for a more realistic way to require the unbalance of the tool system. As mentioned, the unbalance requirements are derived from the amount of load that actually affects the bearings in the spindle which is the base for the entire standard. Following is the fundamental equation for ISO 16084 standard.

Step-by-step calculation guide

1. Identify which spindle interface that applies (has individual defined parameters). E.g. Coromant Capto® C4 or HSK-A63
2. Determine what kind of machining that is to be done
   • Standard machining (f_bal = 0.8)
   • Fine machining (f_bal = 0.2)
3. Input specific parameters for the tool
   • Tool mass (m_t)
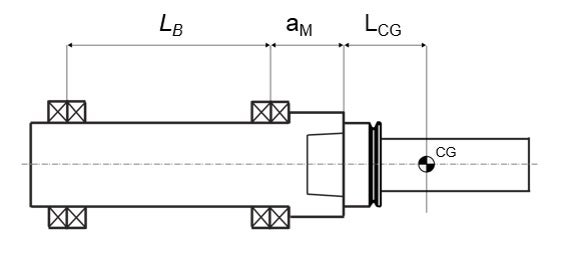
   • Length to centre-of-gravity (L_cg)
   • Rotational speed of the tool in RPM (n)
4. Calculate the maximum allowed unbalance (U_STAT,PER)

Calculation example ISO 16084

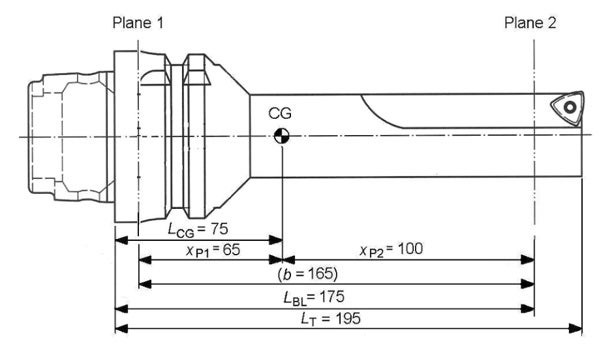
1. Identify spindle interface: HSK-A63
   • C_dyn = 25 000 N (maximum bearing load)
   • A_m = 50 mm (spindle nose bearing)
   • L_b = 415 mm (length between bearings)
   • E_s = 2.00 µm (joining inaccuracy tool shank)
   • U_bm,tol = 0.75 g.mm (tolerance of balancing machine)
2. Machining determined: Fine machining, f_bal = 0.2
3. Specific parameters
   • m_t = 1.4 kg
   • Rotational speed, n=3500 rpm
   • L_cg = 75 mm
4. U_STAT,PER = 282 g.mm